 por GABRIELA » Ter Set 01, 2009 14:15
por GABRIELA » Ter Set 01, 2009 14:15
Estou com a seguinte questão sobre matriz :
A =

Então, calculando-se (a+b)², obtém-se quanto?
Então fiz ( a+b)² = (a+b).(a+b)
Meu resultado deu

E no livro diz que a resposta é

Onde estou errando ?
-
GABRIELA
- Usuário Parceiro

-
- Mensagens: 73
- Registrado em: Seg Ago 31, 2009 17:31
- Formação Escolar: ENSINO MÉDIO
- Andamento: cursando
 por Molina » Ter Set 01, 2009 14:21
por Molina » Ter Set 01, 2009 14:21
GABRIELA escreveu:Estou com a seguinte questão sobre matriz :
A =

Então, calculando-se (a+b)², obtém-se quanto?
Então fiz ( a+b)² = (a+b).(a+b)
Meu resultado deu

E no livro diz que a resposta é

Onde estou errando ?
Boa tarde, Gabriela.
Você terá que fazer:

*

E este elemento que está dando diferente da resposta é dado por:
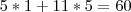
(igual ao gabarito do livro).
Bom estudo,

Diego Molina |
CV |
FB |
.COMEquipe AjudaMatemática.com"Existem 10 tipos de pessoas: as que conhecem o sistema binário e as que não conhecem."
-

Molina
- Colaborador Moderador - Professor

-
- Mensagens: 1551
- Registrado em: Dom Jun 01, 2008 14:10
- Formação Escolar: GRADUAÇÃO
- Área/Curso: Licenciatura em Matemática - UFSC
- Andamento: formado
 por GABRIELA » Ter Set 01, 2009 14:47
por GABRIELA » Ter Set 01, 2009 14:47
molina escreveu:GABRIELA escreveu:Estou com a seguinte questão sobre matriz :
A =

Então, calculando-se (a+b)², obtém-se quanto?
Então fiz ( a+b)² = (a+b).(a+b)
Meu resultado deu

E no livro diz que a resposta é

Onde estou errando ?
Boa tarde, Gabriela.
Você terá que fazer:

*

E este elemento que está dando diferente da resposta é dado por:
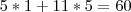
(igual ao gabarito do livro).
Bom estudo,

OK! Entendi como chegar no 60.Estava multiplicando de forma errada.Estva multiplicando coluna x coluna e na verdade é linha x coluna
-
GABRIELA
- Usuário Parceiro

-
- Mensagens: 73
- Registrado em: Seg Ago 31, 2009 17:31
- Formação Escolar: ENSINO MÉDIO
- Andamento: cursando
Voltar para Matrizes e Determinantes
Se chegou até aqui, provavelmente tenha interesse pelos tópicos relacionados abaixo.
Aproveite a leitura. Bons estudos!
-
- [MATRIZ] Como acho o determinante dessa matriz
por LAZAROTTI » Qui Mai 03, 2012 00:38
- 4 Respostas
- 6935 Exibições
- Última mensagem por Russman
Qui Mai 03, 2012 01:56
Matrizes e Determinantes
-
- [Matriz]- inversa de uma matriz
por Ana_Rodrigues » Seg Mar 26, 2012 08:54
- 2 Respostas
- 3406 Exibições
- Última mensagem por Ana_Rodrigues
Seg Mar 26, 2012 18:05
Matrizes e Determinantes
-
- [MATRIZ]Determinante da Matriz 4x4
por LAZAROTTI » Qui Mai 03, 2012 22:33
- 1 Respostas
- 6598 Exibições
- Última mensagem por LuizAquino
Sex Mai 11, 2012 08:00
Matrizes e Determinantes
-
- [Matriz] Matriz com potencias
por rochadapesada » Dom Abr 07, 2013 20:29
- 3 Respostas
- 4535 Exibições
- Última mensagem por DanielFerreira
Seg Abr 08, 2013 17:32
Matrizes e Determinantes
-
- matriz
por Barbara » Ter Ago 18, 2009 15:26
- 4 Respostas
- 4677 Exibições
- Última mensagem por Molina
Qui Ago 20, 2009 18:11
Matrizes e Determinantes
Usuários navegando neste fórum: Nenhum usuário registrado e 1 visitante
Assunto:
[Função] do primeiro grau e quadratica
Autor:
Thassya - Sáb Out 01, 2011 16:20
1) Para que os pontos (1,3) e (-3,1) pertençam ao grafico da função f(X)=ax + b ,o valor de b-a deve ser ?
2)Qual o maior valor assumido pela função f : [-7 ,10] em R definida por f(x) = x ao quadrado - 5x + 9?
3) A função f, do primeiro grau, é definida pos f(x)= 3x + k para que o gráfico de f corte o eixo das ordenadas no ponto de ordenada 5 é?
Assunto:
[Função] do primeiro grau e quadratica
Autor:
Neperiano - Sáb Out 01, 2011 19:46
Ola
Qual as suas dúvidas?
O que você não está conseguindo fazer?
Nos mostre para podermos ajudar
Atenciosamente
Assunto:
[Função] do primeiro grau e quadratica
Autor:
joaofonseca - Sáb Out 01, 2011 20:15
1)Dados dois pontos A=(1,3) e B=(-3,1) de uma reta, é possivel definir a sua equação.
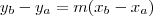
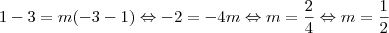
Em

substitui-se
m, substitui-se
y e
x por um dos pares ordenados, e resolve-se em ordem a
b.
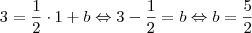
2)Na equação

não existem zeros.Senão vejamos
Completando o quadrado,
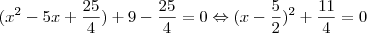
As coordenadas do vertice da parabola são
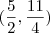
O eixo de simetria é a reta
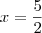
.Como se pode observar o vertice está acima do eixo Ox, estando parabola virada para cima, o vertice é um mínimo absoluto.Então basta calcular a função para os valores dos extremos do intervalo.
f(-7)=93
f(10)=59
Powered by phpBB © phpBB Group.
phpBB Mobile / SEO by Artodia.








 *
* 
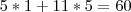 (igual ao gabarito do livro).
(igual ao gabarito do livro).

*
(igual ao gabarito do livro).

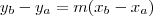
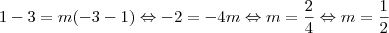
 substitui-se m, substitui-se y e x por um dos pares ordenados, e resolve-se em ordem a b.
substitui-se m, substitui-se y e x por um dos pares ordenados, e resolve-se em ordem a b.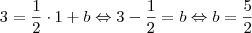
 não existem zeros.Senão vejamos
não existem zeros.Senão vejamos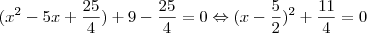
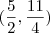
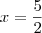 .Como se pode observar o vertice está acima do eixo Ox, estando parabola virada para cima, o vertice é um mínimo absoluto.Então basta calcular a função para os valores dos extremos do intervalo.
.Como se pode observar o vertice está acima do eixo Ox, estando parabola virada para cima, o vertice é um mínimo absoluto.Então basta calcular a função para os valores dos extremos do intervalo.